 Image via WikipediaBenoît Mandelbrot
Image via WikipediaBenoît Mandelbrot, who discovered the mathematical shapes known as fractals, died of cancer, aged 85, on 14 October 2010.
The visionary mathematician was born in Poland into a Jewish family that moved to Paris when he was 11 to escape the Nazis. He subsequently moved to the US and spent most of his life working for IBM. He eventually became a professor of mathematical science at Yale University.
During the 1970s, Mandelbrot developed a new mathematical way of understanding the infinite complexity of nature. In 1975, he introduced a new word to the world: fractal. Mandelbrot's seminal works, Fractals: Form, Chance and Dimension and The
Fractal Geometry of Nature, were published in 1977 and 1982. In these, he showed that seemingly random mathematical shapes in fact often followed a pattern, made of ever smaller, repeating shapes.
So what are
fractals? And why are they important? There is no simple definition of fractals that can be considered truly accurate, but The McGraw-Hill Concise Encyclopedia of Science and Technology defines them thus:
Geometrical objects that are self-similar when the distance at which they are viewed is changed ... The concept is helpful in allowing order to be perceived in apparent disorder, e.g. in the case of a river and its tributaries, every tributary has its own tributaries ... so that it has the same structure and organisation as the entire river except that it covers a smaller area ...
Fractal mathematics is closely related to chaos theory. The best way to get a feeling for fractals is to consider some examples: mountains, coastlines, cauliflowers and ferns are all natural fractals. The concept of the small piece resembling the whole object has allowed scientists to measure the areas and volumes of complicated, irregular objects, including the coastline of Britain, the size of a lung or a cauliflower. They are the sort of shape that mathematicians once shied away from in favour of regular spheres, cones and the like.
Clouds are another classically fractal natural phenomenon. In fact, fractal theory has myriad applications in physics, biology and astronomy. "If you cut one of the florets of a cauliflower, you see the whole cauliflower but smaller," Mandelbrot explained at the Technology Entertainment and Design (TED) conference in California earlier this year. "Then you cut again, again, again, and you still get small cauliflowers. So there are some shapes which have this peculiar property, where each part is like the whole, but smaller."
He also famously wrote: "Clouds are not spheres, mountains are not cones, coastlines are not circles, and bark is not smooth, nor does lightning travel in a straight line." He used the word "roughness" to describe this chaos and irregularity inherent in the natural world.
In fact, although the cauliflower and clouds are famous examples of natural fractals, most things in nature are fractal. A sand dune features ripples that resemble the entire dune; a river system has tributaries that resemble the main river, and each of these has its own tributaries. The closer you look, the more you see the same patterns repeating. The whole universe is fractal, with "self-similarity" at all different scales, the defining characteristic of fractals.
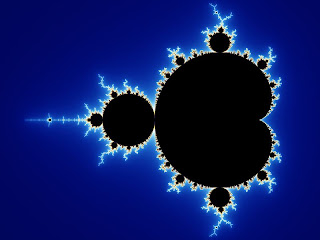 |
The infinitely complex Mandelbrot Set
Created by Wolfgang Beyer with the program Ultra Fractal 3 |
Like many others, I first became aware of fractals in the 1980s. At school I saw a video on the subject produced by Sheffield University, taking a tour of the lurid, infinitely complex
Mandelbrot Set, named in Mandelbrot's honour. At home, I generated my own version of it on my home computer, the single still image taking 20 minutes to appear pixel-by-pixel on the monochrome screen. Now, advances in computing power have allowed us to see the Mandelbrot Set in immense depth. Take a look at this
awe-inspiring video of the Mandelbrot Set, generated by the fractal software Fractice. The familiar shapes of the Mandelbrot Set appear again and again, ghostlike, uninvited.
Few people realise how much fractals have revolutionised our understanding of the world, and how many fractal-based systems we depend upon. The fractal mathematics Mandelbrot pioneered, together with the related field of chaos theory and the advent of computers, unveil these hidden beauties of the natural world. It inspired scientists in many fields - including cosmology, medicine, engineering and genetics - and artists, including those producing remarkably realistic computer graphics. Most well-known are the fractal images, which have inspired countless intricate pieces of art.
Fractal geometry has many practical uses. It can provide a way to understand complexity in "systems" as well as in shapes. in the architecture of the networks comprising the internet, the timing and sizes of earthquakes and the variation in a person's heartbeat and the prevalence and diagnosis of certain diseases. Fractal mathematics has also been applied to such technological developments as digital music and in computer file compression systems.
Another application of fractal theory is in the financial markets, a field Mandelbrot first worked in while studying the mathematics of complexity, working as a researcher for IBM during the 1960s. Mandelbrot applied fractal mathematics to market behaviour, in particular the profits and losses made by traders over time. Mandelbrot returned to this idea in 2005. His book "The (Mis)Behaviour of Markets" sets out a highly critical attack on the world of banking and financial systems, he stressed that the economic models in use were unable to cope with their own complexity, and he warned against the huge risks being taken by traders, who, he claimed, tend to act as if the market is inherently predictable, immune to sudden shocks or changes.
Fractal mathematics cannot be used to predict individual big events in chaotic systems, but it tells us that such events will happen. As such, it reminds us that the world is complex at every scale, and inherently unpredictable.











